Solved Examples on Rational Functions

For ACT Students
The ACT is a timed exam...60 questions for 60 minutes
This implies that you have to solve each question in one minute.
Some questions will typically take less than a minute a solve.
Some questions will typically take more than a minute to solve.
The goal is to maximize your time. You use the time saved on those questions you solved in less than a minute, to solve the questions that will take more than a minute.
So, you should try to solve each question correctly and timely.
So, it is not just solving a question correctly, but solving it correctly on time.
Please ensure you attempt all ACT questions.
There is no negative penalty for a wrong answer.

For WASSCE Students
Any question labeled WASCCE is a question for the WASCCE General Mathematics
Any question labeled WASSCE-FM is a question for the WASSCE Further Mathematics/Elective Mathematics

For GCSE Students
All work is shown to satisfy (and actually exceed) the minimum for awarding method marks.
Calculators are allowed for some questions. Calculators are not allowed for some questions.

For NSC Students
For the Questions:
Any space included in a number indicates a comma used to separate digits...separating multiples of three digits from behind.
Any comma included in a number indicates a decimal point.
For the Solutions:
Decimals are used appropriately rather than commas
Commas are used to separate digits appropriately.

Review:
Given a Rational Function:
To Find the Vertical Asymptote (VA):
(1.) Simplify the function
(2.) Set the denominator to zero (if applicable after simplifying the function)
(3.) Solve for the value of $x$
(4.) $VA: x = value$

To Find the Horizontal Asymptote (HA):
(1.) Arrange the numerator in standard form
(2.) Arrange the denominator in standard form
(3.) If the degree of the numerator is less than the degree of the denominator, $HA: y = 0$
(4.) If the degree of the numerator is the same as the degree of the denominator,

$HA: y = \dfrac{leading\;\;coefficient\;\;of\;\;the\;\;numerator}{leading\;\;coefficient\;\;of\;\;the\;\;denominator}$

If the degree of the numerator is greater than the degree of the denominator, then onto the Slant Asymptote (or Oblique Asymptote)

To Find the Slant Asymptote (SA):
If the degree of the numerator is greater than the degree of the denominator,

$SA: y = quotient\;\;of\;\;\dfrac{numerator}{denominator}$

To Find the $x-intercept$:
(1.) Set $y = 0$
(2.) Solve for the value of $x$
(3.) $x-intercept = (value, 0)$

To Find the $y-intercept$:
(1.) Set $x = 0$
(2.) Solve for the value of $y$
(3.) $y-intercept = (0, value)$

To Find the domain:
What are the values of the input, x that will not give any output, y
Remove those values.
The set of all the input values that will give results when those input values are substituted in the functions is the domain
For a rational function: the denominator must be non-zero.
No division by zero.

$ f(x) = \dfrac{numerator}{denominator} \\[5ex] denominator \ne 0 \\[3ex] $ (You cannot divide something by nothing, neither can you divide nothing by nothing.)
So, we have to find all the values of x for which the denominator is not zero.

To Find the range:
What are all the likely results that could be got from all those input values?
The set of all those output values that could be got when those input values are substituted in the functions is the range.

Solve all questions.
For any question regarding the domain and the range, express the domain and the range in set notation and interval notation.
Show all work.

(1.) Determine the x-intercept and the y-intercept for the function.

$ f(x) = \dfrac{x + 7}{x^2 + 36} \\[5ex] $

$ f(x) = \dfrac{x + 7}{x^2 + 36} \\[5ex] y = \dfrac{x + 7}{x^2 + 36} \\[5ex] \underline{y-intercept} \\[3ex] Set\;\;x = 0 \;\;and\;\;solve\;\;for\;\;y \\[3ex] y = \dfrac{0 + 7}{0^2 + 36} \\[5ex] y = \dfrac{7}{36} \\[5ex] y-intercept = \left(0, \dfrac{7}{36}\right) \\[5ex] \underline{x-intercept} \\[3ex] Set\;\;y = 0 \;\;and\;\;solve\;\;for\;\;x \\[3ex] 0 = \dfrac{x + 7}{x^2 + 36} \\[5ex] Multiply\;\;both\;\;sides\;\;by\;\; x^2 + 36 \\[3ex] 0 = x + 7 \\[3ex] x + 7 = 0 \\[3ex] x = 0 - 7 \\[3ex] x = -7 \\[3ex] x-intercept = (-7, 0) $

(2.) ACT Consider the graph of the equation $y = \dfrac{3x - 12}{2x - 6}$ in the standard (x, y) coordinate plane.
Which of the following equations represents the vertical asymptote of the graph?

$ F.\:\: x = 2 \\[3ex] G.\:\: x = 3 \\[3ex] H.\:\: x = 4 \\[3ex] J.\:\: x = 6 \\[3ex] K.\:\: x = 12 \\[3ex] $

$ \underline{Vertical\;\;Asymptote:\;VA} \\[3ex] y = \dfrac{3x - 12}{2x - 6} \\[5ex] y = \dfrac{3(x - 4)}{2(x - 3)} \\[5ex] Nothing\;\;cancels \\[3ex] Set\;\;the\;\;denominator\;\;to\;\;0; \;\;\;\;solve\;\;for\;\;x \\[3ex] 2x - 6 = 0 \\[3ex] 2x = 0 + 6 \\[3ex] 2x = 6 \\[3ex] x = \dfrac{6}{2} \\[5ex] x = 3 \\[3ex] VA:\;\;x = 3 $

(3.) Determine the intercepts and the vertical asymptote of

$ f(x) = \dfrac{x^2 - 3x - 4}{x - 5} \\[5ex] $

$ f(x) = \dfrac{x^2 - 3x - 4}{x - 5} \\[5ex] y = \dfrac{x^2 - 3x - 4}{x - 5} \\[5ex] x-intercept:\;\; \\[3ex] set\;\;y = 0; \;\;\;\;solve\;\;for\;\;x \\[3ex] y = \dfrac{x^2 - 3x - 4}{x - 5} \\[5ex] 0 = \dfrac{x^2 - 3x - 4}{x - 5} \\[5ex] 0(x - 5) = x^2 - 3x - 4 \\[3ex] x^2 - 3x - 4 = 0 \\[3ex] (x + 1)(x - 4) = 0 \\[3ex] x + 1 = 0 \;\;OR\;\;x - 4 = 0 \\[3ex] x = -1 \;\;OR\;\; x = 4 \\[3ex] x-intercepts\;\;are:\;\; (-1, 0) \;\;and\;\; (4, 0) \\[3ex] y-intercept:\;\; \\[3ex] set\;\;x = 0; \;\;\;\;solve\;\;for\;\;y \\[3ex] y = \dfrac{x^2 - 3x - 4}{x - 5} \\[5ex] y = \dfrac{0^2 - 3(0) - 4}{0 - 5} \\[5ex] y = \dfrac{0 - 0 - 4}{-5} \\[5ex] y = \dfrac{-4}{-5} \\[5ex] y = \dfrac{4}{5} \\[5ex] y-intercept\;\;is:\;\; \left(0, \dfrac{4}{5}\right) \\[5ex] \underline{Vertical\;\;Asymptote:\;VA} \\[3ex] y = \dfrac{x^2 - 3x - 4}{x - 5} \\[5ex] y = \dfrac{(x + 1)(x - 4)}{x - 5} \\[5ex] Nothing\;\;cancels \\[3ex] set\;\;denominator\;\;to\;\;0; \;\;\;\;solve\;\;for\;\;x \\[3ex] x - 5 = 0 \\[3ex] x = 5 \\[3ex] VA:\;\;x = 5 $

(4.) Fill in the dots.
If, as x → ∞, the values of R(x) approach some fixed number L, then the line y = L is a ............ of the graph of R.

If, as x → ∞, the values of R(x) approach some fixed number L, then the line y = L is a horizontal asympote of the graph of R.

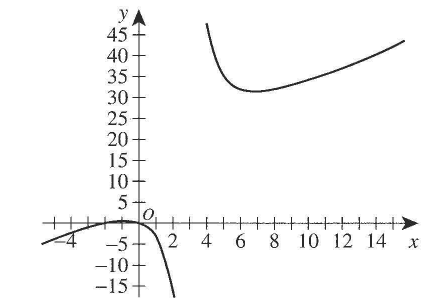
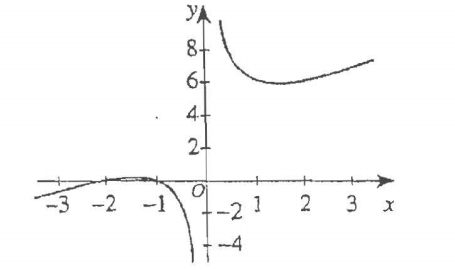
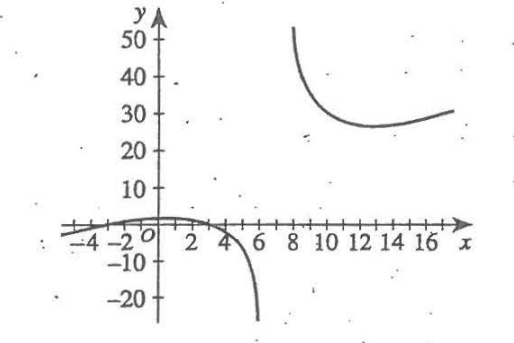
(5.) ACT The graph below shows the function $f(x) = \dfrac{2x - 5}{7x + 5}$ in the standard (x, y) coordinate plane.
Which of the following is an equation of the horizontal asymptote of f(x)?

$ F.\;\; x = -1 \\[3ex] G.\;\; x = \dfrac{2}{7} \\[5ex] H.\;\; y = -1 \\[3ex] J.\;\; y = \dfrac{2}{7} \\[5ex] K.\;\; y = \dfrac{5}{7} \\[5ex] $

$ f(x) = \dfrac{2x - 5}{7x + 5} \\[5ex] \underline{Horizontal\;\;Asymptote:\;HA} \\[3ex] numerator\;\;in\;\;standard\;\;form ...yes \\[3ex] denominator\;\;in\;\;standard\;\;form ... yes \\[3ex] Degree\;\;of\;\;Numerator = Degree\;\;of\;\;Denominator = 1 \\[3ex] HA: y = \dfrac{leading\;\;coefficient\;\;of\;\;the\;\;numerator}{leading\;\;coefficient\;\;of\;\;the\;\;denominator} \\[5ex] HA: y = \dfrac{2}{7} $

(6.) ACT In the standard (x, y) coordinate plane, the graph of which of the following equations has the line $x = 2$ as a vertical asymptote?

$ A.\;\; y = \dfrac{4x + 3}{2x} \\[5ex] B.\;\; y = \dfrac{4x + 3}{x + 2} \\[5ex] C.\;\; y = \dfrac{4x + 3}{x - 2} \\[5ex] D.\;\; y = \dfrac{x + 2}{4x + 3} \\[5ex] E.\;\; y = \dfrac{x - 2}{4x + 3} \\[5ex] $

To determine the vertical asymptote:
(1.) Simplify the function
(2.) Set the denominator to zero
(3.) Solve for $x$

$ All\;\;the\;\;functions\;\;are\;\;already\;\;simplified \\[3ex] Looking\;\;at\;\;the\;\;denominators: \\[3ex] x - 2 = 0 \\[3ex] x = 2 \\[3ex] Correct\;\;Option:\;\; C $

(7.) ACT At what point in the standard (x, y) coordinate plane asymptotes of the function $y = \dfrac{2x(x + 2)}{x - 3}$, graphed below, intersect?

$ F.\;\; \left(-\dfrac{7}{3}, 3\right) \\[5ex] G.\;\; \left(\dfrac{7}{3}, 10\right) \\[5ex] H.\;\; (3, 10) \\[3ex] J.\;\; (3, 16) \\[3ex] K.\;\; (3, 31) \\[3ex] $

Only two asymptotes apply to the function: the Vertical Asymptote (VA) and the Slant Asymptote (SA)
So, we shall find both asymptotes.
Then, we shall answer the question (the intersection of both asymptotes)

$ \underline{Vertical\;\;Asymptote:\;VA} \\[3ex] y = \dfrac{2x(x + 2)}{x - 3} \\[5ex] Nothing\;\;cancels \\[3ex] Set\;\;the\;\;denominator\;\;to\;\;0; \;\;\;\;solve\;\;for\;\;x \\[3ex] x - 3 = 0 \\[3ex] x = 0 + 3 \\[3ex] x = 3 \\[3ex] VA:\;\;x = 3 \\[3ex] \underline{Slant\;\;Asymptote:\;SA} \\[3ex] y = \dfrac{2x(x + 2)}{x - 3} \\[5ex] y = \dfrac{2x^2 + 4x}{x - 3} \\[5ex] y = \dfrac{2x^2 + 4x + 0}{x - 3} \\[5ex] \require{enclose} \begin{array}{rl6} 2x + 10 \\[-3pt] x - 3 \enclose{longdiv}{2x^2 + 4x + 0}\kern-.5ex \\[-3pt] \underline{2x^2 - 6x\phantom{000}} \\[-3pt] 10x + 0\phantom{0} \\[-3pt] \underline{\phantom{0}10x - 30} \\[-3pt] \phantom{0}30 \\[-3pt] \end{array} \\[3ex] SA:\;\; y = 2x + 10 \\[3ex] \underline{Intersection\;\;of\;\;VA\;\;and\;\;SA} \\[3ex] VA:\;\;x = 3 \\[3ex] SA:\;\; y = 2x + 10 \\[3ex] y = 2(3) + 10 \\[3ex] y = 6 + 10 \\[3ex] y = 16 \\[3ex] Intersection:\;\;(x, y) = (3, 16) $

(8.) ACT The graph of the function $f(x) = \dfrac{x^2 + 3x + 2}{x}$ is shown in the standard (x, y) coordinate plane below.
Which of the following, if any, is a list of each of the vertical asymptotes of $f(x)$?

$ F.\;\; x = 0 \\[3ex] G.\;\; x = -1 \;\;and\;\; x = -2 \\[3ex] H.\;\; y = x + 3 \\[3ex] J.\;\; y = 3x + 2 \\[3ex] K.\;\; This\;\;function\;\;has\;\;no\;\;vertical\;\;asymptote \\[3ex] $

(9.) Determine the domain and range of the function: $xy = -7$

$ xy = -7 \\[3ex] y = -\dfrac{7}{x} \\[5ex] $ It is a function (rational function).
The denominator of rational functions must be non-zero.
We cannot divide anything by nothing.
Dividing anything (something or nothing) by nothing does not give us a real number.
It is undefined.
We are dealing with only real numbers.

$ D = \{x | x \in \mathbb{R}; x \ne 0\} \\[3ex] D = (-\infty, 0) \bigcup (0, \infty) \\[3ex] $ To find the range, let us assume the output is $0$

$ y = -\dfrac{7}{x} \\[5ex] 0 = -\dfrac{7}{x} \\[5ex] -\dfrac{7}{x} = 0 \\[5ex] -7 = x * 0 \\[3ex] -7 = 0? \:This\: is\: a\: contradiction \\[3ex] $ $0$ can never be an output.
Any other number can be an output.

$ R = \{y | y \in \mathbb{R}; y \ne 0\} \\[3ex] R = (-\infty, 0) \bigcup (0, \infty) $

(10.) ACT At what value(s) of x is $\dfrac{(x - 3)^2}{x^2}$ undefined?

F. 0 only
G. 0 and 3 only
H. −3 only
J. −3 and 0 only
K. −3, 0, and 3 only

The function is undefined when the denominator is zero.
In other words, find the vertical asymptote of the function.

$ \underline{Vertical\;\;Asymptote:\;VA} \\[3ex] f(x) = \dfrac{(x - 3)^2}{x^2} \\[5ex] Nothing\;\;cancels \\[3ex] Set\;\;the\;\;denominator\;\;to\;\;0; \;\;\;\;solve\;\;for\;\;x \\[3ex] x^2 = 0 \\[3ex] x = \pm \sqrt{0} \\[3ex] x = \pm 0 \\[3ex] x = 0 \\[3ex] VA:\;\;x = 0 $

(11.) Determine the domain and range of the function: $y = 9 - \dfrac{7}{x}$

It is a function (rational function).
The denominator of rational functions must be non-zero.
We cannot divide anything by nothing.
Dividing anything (something or nothing) by nothing does not give us a real number.
It is undefined.
We are dealing with only real numbers.

$ D = \{x | x \in \mathbb{R}; x \ne 0\} \\[3ex] D = (-\infty, 0) \bigcup (0, \infty) \\[3ex] $ To find the range, let us assume the output is $0$

$ y = 9 - \dfrac{7}{x} \\[5ex] 0 = 9 - \dfrac{7}{x} \\[5ex] \dfrac{7}{x} = 9 \\[5ex] 7 = 9x \\[3ex] 9x = 7 \\[3ex] x = \dfrac{7}{9} \\[5ex] $ An input of $\dfrac{7}{9}$ will give an output of $0$.

So, $0$ can be an output.

What do you think we should do?

Let us assume the output is $9$

$ y = 9 - \dfrac{7}{x} \\[5ex] 9 = 9 - \dfrac{7}{x} \\[5ex] \dfrac{7}{x} = 9 - 9 \\[5ex] \dfrac{7}{x} = 0 \\[5ex] 7 = x * 0 \\[3ex] 7 = 0? \:\:\:This\:\:\: is\:\:\: a\:\:\: contradiction \\[3ex] $ $9$ can never be an output.
Any other number can be an output.

$ R = \{y | y \in \mathbb{R}; y \ne 9\} \\[3ex] R = (-\infty, 9) \bigcup (9, \infty) \\[3ex] $

(12.) ACT The equation $y = \dfrac{2x^2 - 18}{x^2 - 5x}$ has 2 vertical asymptotes and 1 horizontal asymptote.
What is the horizontal asymptote?

$ F.\;\; x = 0 \\[3ex] G.\;\; x = 3 \\[3ex] H.\;\; x = 9 \\[3ex] J.\;\; y = 0 \\[3ex] K.\;\; y = 2 \\[3ex] $

$ y = \dfrac{2x^2 - 18}{x^2 - 5x} \\[5ex] \underline{Horizontal\;\;Asymptote:\;HA} \\[3ex] numerator\;\;in\;\;standard\;\;form ...yes \\[3ex] denominator\;\;in\;\;standard\;\;form ... yes \\[3ex] Degree\;\;of\;\;Numerator = Degree\;\;of\;\;Denominator = 2 \\[3ex] HA: y = \dfrac{leading\;\;coefficient\;\;of\;\;the\;\;numerator}{leading\;\;coefficient\;\;of\;\;the\;\;denominator} \\[5ex] HA: y = \dfrac{2}{1} \\[5ex] HA: y = 2 $

(13.) Determine the domain and range of the function: $y = \dfrac{5}{x - 4}$

It is a function (rational function).
The denominator of rational functions must be non-zero.

$ x - 4 \ne 0 \\[3ex] x \ne 4 \\[3ex] D = \{x | x \in \mathbb{R}; x \ne 4\} \\[3ex] D = (-\infty, 4) \bigcup (4, \infty) \\[3ex] $ To find the range, let us assume the output is $0$

$ y = \dfrac{5}{x - 4} \\[5ex] 0 = \dfrac{5}{x - 4} \\[5ex] \dfrac{5}{x - 4} = 0 \\[5ex] 5 = (x - 4) * 0 \\[3ex] 5 = 0? \:This\: is\: a\: contradiction \\[3ex] $ $0$ can never be an output.
Any other number can be an output.

$ R = \{y | y \in \mathbb{R}; y \ne 0\} \\[3ex] R = (-\infty, 0) \bigcup (0, \infty) $

(14.) Fill in the dots.
If, as x approaches some number c, the values of |R(x)| → ∞, then the line x = c is a ............ of the graph of R.

If, as x approaches some number c, the values of |R(x)| → ∞, then the line x = c is a vertical asympote of the graph of R.

(15.) What is the value of $f(x)$ at x = 4?

$ F.\;\; 4 \\[3ex] G.\;\; 2 \\[3ex] H.\;\; \dfrac{5}{3} \\[5ex] J.\;\; -1 \\[3ex] K.\;\; -\dfrac{7}{3} \\[5ex] $

$ f(x) = \dfrac{x^2 - 9}{x - 7} \\[5ex] When\;\; x = 4 \\[3ex] f(4) \\[3ex] = \dfrac{x^2 - 9}{x - 7} \\[5ex] = \dfrac{4^2 - 9}{4 - 7} \\[5ex] = \dfrac{16 - 9}{-3} \\[5ex] = \dfrac{7}{-3} \\[5ex] = -\dfrac{7}{3} $

(16.) What is the domain of $f(x)$?
(Note: The domain of a function is all the $x-values$ for which the function is defined.)

A. All real values of $x$ except $\pm 3$

B. All real values if $x$ except $\dfrac{9}{7}$

C. All real values of $x$ except $7$

D. All real values of $x$ except $\pm 3$ and $7$

E. All real values of $x$ where $x \le -6$ or $x \ge 8$

In this case: (based on this question):
Th denominator cannot be zero
Why?
Because division by zero is undefined
Therefore, to find the domain, set the denominator to zero; and exclude the value of $x$ that makes the denominator to be zero

$ x - 7 = 0 \\[3ex] x = 0 + 7 \\[3ex] x = 7 \\[3ex] $ Therefore, the domain is the set of all the real values of $x$ except $7$

(17.) How many horizontal and/or vertical asymptotes are there for the graph of $f(x)$?

$ F.\;\; 4 \\[3ex] G.\;\; 3 \\[3ex] H.\;\; 2 \\[3ex] J.\;\; 1 \\[3ex] K.\;\; 0 \\[3ex] $

To determine the vertical asymptote:
(1.) Simplify the function
(2.) Set the denominator to zero
(3.) Solve for $x$

$ \underline{Vertical\;\;Asymptote:\;VA} \\[3ex] f(x) = \dfrac{x^2 - 9}{x - 7} \\[5ex] f(x) = \dfrac{x^2 - 3^2}{x - 7} \\[5ex] f(x) = \dfrac{(x + 3)(x - 3)}{x - 7} \\[5ex] Nothing\;\;cancels \\[3ex] Set\;\;the\;\;denominator\;\;to\;\;0; \;\;\;\;solve\;\;for\;\;x \\[3ex] x - 7 = 0 \\[3ex] x = 7 \\[3ex] VA:\;\;x = 7...only\;\;1\;\;vertical\;\;asymptote \\[5ex] \underline{Horizontal\;\;Asymptote:\;HA} \\[3ex] numerator\;\;in\;\;standard\;\;form ...yes \\[3ex] denominator\;\;in\;\;standard\;\;form ... yes \\[3ex] Degree\;\;of\;\;Numerator = 2 \\[3ex] Degree\;\;of\;\;Denominator = 1 \\[3ex] $ Because the Degree of the Numerator is greater than the Degree of the Denominator, there is no horizontal asymptote.
In this case, we have a slant asymptote.
Therefore, we have only 1 vertical asymptote.
The correct option is J.

(18.) ACT The expression $\dfrac{2b + c}{b - 2c}$ is undefined whenever b = ?

$ F.\;\; -2c \\[3ex] G.\;\; -\dfrac{1}{2}c \\[5ex] H.\;\; 0 \\[3ex] J.\;\; \dfrac{1}{2}c \\[5ex] K.\;\; 2c \\[3ex] $

The expression $\dfrac{2b + c}{b - 2c}$ is undefined when the denominator is zero
Set the denominator to zero and solve for b

$ b - 2c = 0 \\[3ex] b = 0 + 2c \\[3ex] b = 2c $

(19.) Determine the domain and range of the function: $y = -\dfrac{4}{2x + 5}$

It is a function (rational function).
The denominators of rational functions must be non-zero.

$ 2x + 5 \ne 0 \\[3ex] 2x \ne -5 \\[3ex] x \ne -\dfrac{5}{2} \\[5ex] D = \{x | x \in \mathbb{R}; x \ne -\dfrac{5}{2}\} \\[5ex] D = \left(-\infty, -\dfrac{5}{2}\right) \bigcup \left(-\dfrac{5}{2}, \infty\right) \\[5ex] $ To find the range, let us assume the output is $0$

$ y = -\dfrac{4}{2x + 5} \\[5ex] 0 = -\dfrac{4}{2x + 5} \\[5ex] -\dfrac{4}{2x + 5} = 0 \\[5ex] -4 = (2x + 5) * 0 \\[3ex] -4 = 0? \:This\: is\: a\: contradiction \\[3ex] $ $0$ can never be an output.
Any other number can be an output.

$ R = \{y | y \in \mathbb{R}; y \ne 0\} \\[3ex] R = (-\infty, 0) \bigcup (0, \infty) $

(20.) ACT Which of the following lists the real values of x that make the expression $\dfrac{x + 1}{x^2 - 2x - 3}$ undefined?

F. 0 only
G. 3 only
H. -1, 3 only
J. 1, -3 only
K. 0, 1, -3 only

The expression $\dfrac{x + 1}{x^2 - 2x - 3}$ is undefined when the denominator is zero
Set the denominator to zero and solve for x

$ x^2 - 2x - 3 = 0 \\[3ex] (x + 1)(x - 3) = 0 \\[3ex] x + 1 = 0 \;\;OR\;\; x - 3 = 0 \\[3ex] x = -1 \;\;OR\;\; x = 3 \\[3ex] -1, \;\;3\;\;only $

(21.) Determine the domain and range of the function: $f(x) = \dfrac{1}{x^2 - x - 6}$

It is a rational function.
First, let us deal with the denominator.
The denominator should not be equal to zero.
The denominator is quadratic. It has two roots.
So, we have to find those two roots.
The denominator should not be equal to those roots
Those roots should not be in our domain because it will give a denominator of zero.
A denominator of zero is an undefined function.

$ x^2 - x - 6 \ne 0 \\[3ex] (x + 2)(x - 3) \ne 0 \\[3ex] x + 2 \ne 0 \:\:\:OR\:\:\: x - 3 \ne 0 \\[3ex] x \ne -2 \:\:\:OR\:\:\: x \ne 3 \\[3ex] $ Second, let us deal with the numerator.
The numerator is just $1$. It is a constant.
That is not a problem.
So, our domain will include all real numbers besides $-2$ and $3$

$ D = \{x | x \ne -2, x \ne 3\} \\[3ex] D = (-\infty, -2) \bigcup (-2, 3) \bigcup (3, \infty) \\[3ex] $ To find the range, we have to be "extra careful" here
First, let us assume our output to be $0$

$ y = \dfrac{1}{x^2 - x - 6} \\[5ex] 0 = \dfrac{1}{x^2 - x - 6} \\[5ex] \dfrac{1}{x^2 - x - 6} = 0 \\[5ex] 1 = (x^2 - x - 6) * 0 \\[3ex] 1 = 0? \:This\: is\: a\: contradiction \\[3ex] $ $0$ can never be an output.

Second, let us note that we should only get real numbers as our results.
Consider the fact that the denominator is quadratic.
The discriminant should not be negative because it will lead to complex number results.
So, let us calculate the discriminant.

$ x^2 - x - 6 \\[3ex] Compare:\:\:\: ax^2 + bx + c \\[3ex] a = 1, b = -1, c = -6 \\[3ex] Discriminant = b^2 - 4ac \\[3ex] = (-1)^2 - 4(1)(-6) \\[3ex] = 1 + 24 \\[3ex] = 25 \\[3ex] R = \{y | y \le -\dfrac{4}{25} \:\:\:OR\:\:\: y \gt 0\} \\[3ex] R = \left(-\infty, -\dfrac{4}{25}\right) \bigcup (0, \infty) $

(22.) ACT For what values of x is the expression $\dfrac{1}{x^2 - 4}$ undefined?

$ F.\;\; -4 \;\;and\;\; 4 \\[3ex] G.\;\; -2 \;\;and\;\; 2 \\[3ex] H.\;\; -1 \;\;and\;\; 1 \\[3ex] J.\;\; -\dfrac{1}{2} \;\;and\;\; \dfrac{1}{2} \\[5ex] K.\;\; -\dfrac{1}{4} \;\;and\;\; \dfrac{1}{4} \\[5ex] $

$\dfrac{1}{x^2 - 4}$ is undefined when the denominator is zero

Set the denominator to zero and solve for x

$ x^2 - 4 = 0 \\[3ex] x^2 = 4 \\[3ex] x = \pm \sqrt{4} \\[3ex] x = \pm 2...Option\;G $

(23.) ACT Which of the following linear equations gives the vertical asymptote for the graph of $y = \dfrac{201x + 202}{203x + 204}$ in the standard (x, y) coordinate plane?

$ A.\:\: x = -\dfrac{201}{203} \\[5ex] B.\:\: x = -\dfrac{202}{201} \\[5ex] C.\:\: x = -\dfrac{202}{204} \\[5ex] D.\:\: x = -\dfrac{204}{203} \\[5ex] E.\:\: x = -\dfrac{403}{407} \\[5ex] $

$ \underline{Vertical\;\;Asymptote:\;VA} \\[3ex] y = \dfrac{201x + 202}{203x + 204} \\[5ex] Nothing\;\;cancels \\[3ex] Set\;\;the\;\;denominator\;\;to\;\;0; \;\;\;\;solve\;\;for\;\;x \\[3ex] 203x + 204 = 0 \\[3ex] 203x = 0 - 204 \\[3ex] 203x = -204 \\[3ex] x = -\dfrac{204}{203} \\[5ex] VA:\;\;x = -\dfrac{204}{203} $

(24.) NYSED Regents Examination The function $f(x) = \dfrac{x - 3}{x^2 + 2x - 8}$ is undefined when x equals

(1) 2 or −4 (3) 3, only
(2) 4 or −2 (4) 2, only

The expression $\dfrac{x - 3}{x^2 + 2x - 8}$ is undefined when the denominator is zero
Set the denominator to zero and solve for x

$ x^2 + 2x - 8 = 0 \\[3ex] (x + 4)(x - 2) = 0 \\[3ex] x + 4 = 0 \;\;OR\;\; x - 2 = 0 \\[3ex] x = -4 \;\;OR\;\; x = 2 \\[3ex] 2 \;\;or\;\; -4 $

(26.) USSCE: Advance Mathematics Paper 1 The graph of the rational function $y = \dfrac{x + 3}{x - 2}$ has a

A. vertical asymptote at x = −3
B. horizontal asymptote at y = 2
C. vertical asymptote at x = 2
D. horizontal asymptote at x = −3

To determine the vertical asymptote:
(1.) Simplify the function
(2.) Set the denominator to zero
(3.) Solve for $x$

$ \underline{Vertical\;\;Asymptote:\;VA} \\[3ex] y = \dfrac{x + 3}{x - 2} \\[5ex] Already\;\;simplified \\[3ex] Set\;\;the\;\;denominator\;\;to\;\;0; \;\;\;\;solve\;\;for\;\;x \\[3ex] x - 2 = 0 \\[3ex] x = 2 \\[3ex] VA:\;\;x = 2 \\[5ex] \underline{Horizontal\;\;Asymptote:\;HA} \\[3ex] numerator\;\;in\;\;standard\;\;form ...yes \\[3ex] denominator\;\;in\;\;standard\;\;form ... yes \\[3ex] Degree\;\;of\;\;Numerator = Degree\;\;of\;\;Denominator = 1 \\[3ex] HA: y = \dfrac{leading\;\;coefficient\;\;of\;\;the\;\;numerator}{leading\;\;coefficient\;\;of\;\;the\;\;denominator} \\[5ex] HA: y = \dfrac{1}{1} \\[5ex] HA: y = 1 \\[3ex] $ The correct option is: C

(28.) ACT In the standard (x, y) coordinate plane, when $a \ne 0$ and $b \ne 0$, the graph of $f(x) = \dfrac{2x + b}{x + a}$ has a horizontal asymptote at:

$ F.\;\; y = 2 \\[3ex] G.\;\; y = a \\[3ex] H.\;\; y = -a \\[3ex] J.\;\; y = -\dfrac{b}{2} \\[5ex] K.\;\; y = \dfrac{b}{a} \\[5ex] $

$ \underline{Horizontal\;\;Asymptote:\;HA} \\[3ex] numerator\;\;in\;\;standard\;\;form ...yes \\[3ex] denominator\;\;in\;\;standard\;\;form ... yes \\[3ex] Degree\;\;of\;\;Numerator = Degree\;\;of\;\;Denominator = 1 \\[3ex] HA: y = \dfrac{leading\;\;coefficient\;\;of\;\;the\;\;numerator}{leading\;\;coefficient\;\;of\;\;the\;\;denominator} \\[5ex] HA: y = \dfrac{2}{1} \\[5ex] HA: y = 2 $

(32.) ACT As x continually increases in value without bound, the value of $\dfrac{x}{x + 3}$ most closely approaches:

$ F.\;\; 0 \\[3ex] G.\;\; \dfrac{1}{3} \\[5ex] H.\;\; 1 \\[3ex] J.\;\; 3 \\[3ex] K.\;\; \infty \\[3ex] $

The question is asking for the horizontal asympote because that is the definition of horizontal asympote.
What happens to the value of y as x increases without bound, and as x decreases without bound.

$ \underline{Horizontal\;\;Asymptote:\;HA} \\[3ex] numerator\;\;in\;\;standard\;\;form ...yes \\[3ex] denominator\;\;in\;\;standard\;\;form ... yes \\[3ex] Degree\;\;of\;\;Numerator = Degree\;\;of\;\;Denominator = 1 \\[3ex] HA: y = \dfrac{leading\;\;coefficient\;\;of\;\;the\;\;numerator}{leading\;\;coefficient\;\;of\;\;the\;\;denominator} \\[5ex] HA: y = \dfrac{1}{1} \\[5ex] HA: y = 1 \\[3ex] $ As x continually increases in value without bound, the value of $\dfrac{x}{x + 3}$ most closely approaches 1